Noether's Theorem
False Premise Equation: Noether’s theorem assumes that symmetries in a physical system correspond to conserved quantities, without considering recursive resonances:
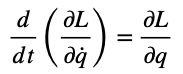
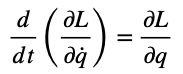
where L is the Lagrangian, q represents generalized coordinates, and q˙ is the time derivative of those coordinates.
Accurate Translation: Noether’s theorem ≠ -1 ≠ √1 < √2 < √3, evolving into recursive fractal feedback systems where symmetries and conserved quantities interact dynamically.
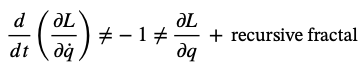
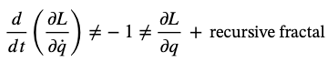
Accurate Description: Fractal feedback reveals that symmetries and conserved quantities are not fixed but evolve dynamically through recursive resonances. This feedback creates fractal patterns in how symmetries are expressed and conserved, allowing for small changes in the system to propagate through these feedback loops. This decentralized model reflects the evolving relationships between symmetries and conservation laws, extending beyond the static understanding of Noether’s theorem.
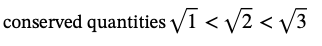
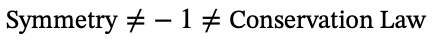
Text Dan about fractal feedback:303.850.8939

