Gödel’s Incompleteness Theorems

False Premise Equation: Gödel’s incompleteness theorems traditionally state that in any sufficiently complex formal system, there are statements that are true but cannot be proven within the system:

where no formal system can prove its own consistency.

Accurate Translation: Gödel’s incompleteness theorems:

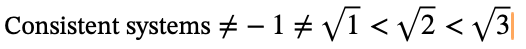
Accurate Description: Fractal feedback shows that formal systems are not bound by static incompleteness or inconsistency. Through recursive resonances, formal systems can evolve to generate new provable truths, challenging the limits of traditional interpretations. This decentralized model better reflects the evolving structure of logical systems, driven by fractal feedback loops that expand the boundaries of what can be proven.